But because Europa is constantly bombarded by intense radiation created by Jupiter’s magnetic field, the Clipper spacecraft can’t orbit the moon itself.
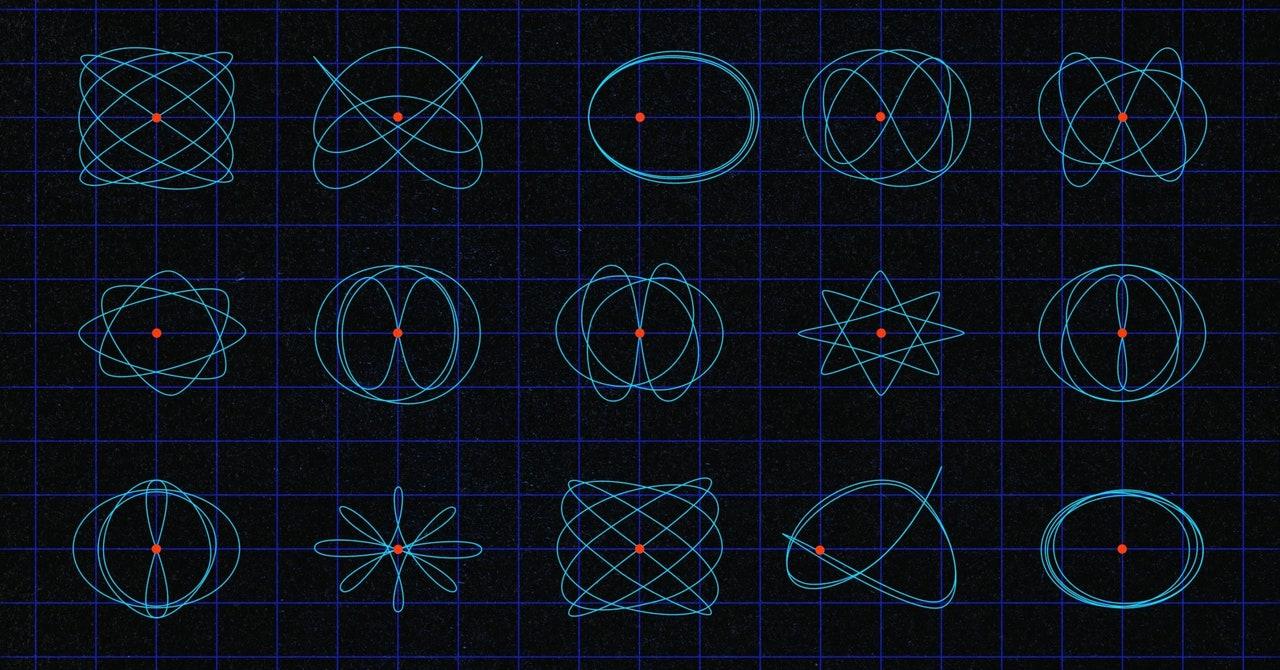
Jovian orbits that are far from Europa form such a family; so do orbits close to Europa.
Spacecraft can orbit this point, even though there is nothing at the center of the orbit.
Some orbit families might require less fuel than others, remain in sunlight at all times, or have other useful features.
The cylinder, along with the laws that determine the paths the pendulum can follow, forms a symplectic space.
To describe the motion of three bodies, like Jupiter, Europa and a spacecraft, you need 18 dimensions: six per body.
The symplectic space only has to account for the spacecraft’s position and velocity, since Jupiter and Europa’s motion can be easily described.
So instead of being 18-dimensional, the corresponding symplectic space is six-dimensional.
This story was originally published in Quanta Magazine.
NASA’s Europa Clipper mission is set to be launched in October by a Falcon Heavy rocket from Cape Canaveral in Florida. Europa is the fourth-largest moon of Jupiter; the $5 billion mission’s goal is to determine whether life exists there. However, the Clipper spacecraft is unable to orbit the moon itself because Europa is continuously hit by powerful radiation produced by Jupiter’s magnetic field. Rather, it will enter an elliptical orbit around Jupiter and collect data by swinging by Europa 53 times in total before pulling back from the strongest radiation. To ensure that it can take images and collect data from Europa’s poles to equator, the spacecraft’s path will vary slightly each time it circles Jupiter.
Trajectory planners use computer models that painstakingly calculate the trajectory one step at a time in order to plan intricate tours such as this one. Decades of mathematical study into orbits and how to connect them into intricate tours have strengthened the planning, which takes hundreds of mission requirements into consideration. A more systematic understanding of the relationships between orbits is being developed by mathematicians through the development of new tools.
Our past calculations serve as a guide for the current calculations that we are performing. However, it’s not a comprehensive representation of all the options available to us,” University of Colorado, Boulder aerospace engineer Daniel Scheeres said.
According to Dayung Koh, an engineer at NASA’s Jet Propulsion Laboratory, “I think that was my biggest frustration when I was a student.”. Although I’m not sure why, I am aware that these orbits exist. It’s problematic to not understand why orbits are where they are given the cost and difficulty of missions to Jupiter’s and Saturn’s moons. As Koh stated: “Did I find them all? Are there more? I can’t tell that.” What if there is an entirely different orbit that could accomplish the task with fewer resources? “.
Following her doctorate from the University of Southern California in 2016, Koh developed a keen interest in the process of classifying orbits into families. Both distant and near-European Jupiterian orbits belong to this family. Other families, though, are less noticeable. For example, there exists an intermediate point where the gravitational effects of any two bodies, such as Jupiter and Europa, balance to form stable points. Though there is nothing at the center of the orbit, spacecraft are still able to circle this point. These orbits are members of a family known as Lyapunov orbits. To initially remain in the same family, add a little energy to such an orbit by starting the spacecraft’s engine. However, if you add more, you’ll enter a different family, such as one that has Jupiter within its orbits. Certain orbit families may have other beneficial characteristics, use less fuel than others, or always be in the sun.
Koh came upon a paper in 2021 that addressed chaotic orbits from the viewpoint of symplectic geometry, an abstract branch of mathematics that is typically kept apart from the messy details of the real world. She contacted the paper’s author, Agustin Moreno, after beginning to suspect that symplectic geometry might provide her with the resources she needed to comprehend orbits more fully. Astonished to learn that someone at NASA was considering his work, Moreno, who was a postdoctoral fellow at Uppsala University in Sweden at the time, felt happy and relieved. He said, “It was unexpected, but it was also pretty interesting and kind of motivating at the same time.”.
Together, they set about applying Moreno’s abstract methods to the Jupiter-Europa system, as well as to Saturn and its moon Enceladus, which, like Europa, may harbor life in its subterranean ocean. Together with other collaborators, they have authored several papers in the past year that establish a framework for orbit cataloging. After completing an early draft in January, Moreno—who is currently a professor at Heidelberg University—turned his survey paper into a book on the topic. Through the book, he hopes to help engineers planning space missions better understand the abstract field of symplectic geometry. He will be bringing together academic disciplines that have drifted apart over the ages if he is successful.
Geometry has no Royal Road.
Physics is the source of symplectic geometry. Consider a pendulum as a basic illustration. Two characteristics of its motion are its angle and its speed. A sufficiently low speed will cause the pendulum to oscillate back and forth. It will rotate in a circle if the speed is greater. The system’s behavior is fixed for all time in an idealized pendulum with no friction once the starting angle and speed are selected.
The angle and speed can be plotted as the x- and y-axes, respectively, on a graph. You can, however, sew together the vertical lines where x is zero degrees and where x is 360 degrees because traveling 360 degrees returns you to the starting point. This results in a cylinder. The cylinder depicts the states of the pendulum at each point rather than a direct representation of the physical world. It does not show the paths that the pendulum follows. There is a symplectic space formed by the cylinder and the laws governing the possible paths the pendulum can take.
Scientists and mathematicians have a firm grasp on how to describe the motion of two bodies subject to gravity since the early 17th century, when Johannes Kepler formulated his laws. Their paths take the shape of an ellipse, a parabola, or a hyperbola, depending on how quickly they are traveling. The corresponding symplectic spaces are tractable, although they are more complex than the one for a pendulum. However, it is impossible to calculate precise, analytical solutions when a third object is added. And when you include more bodies in the model, it only becomes more intricate. At some point, Scheeres claimed, “you’re shooting into the dark without that analytic insight.”.
Three coordinates are required to describe a spacecraft’s position, and three more are needed to describe its velocity, if the spacecraft is free to move in all directions (right to left, up and down, and front to back). Thus, a six-dimensional symplectic space is created. Six dimensions are required for each of the three bodies—Jupiter, Europa, and a spacecraft—to be described in order to explain their motion. The curves that illustrate the evolution of the physical system under description over time, in addition to the number of dimensions, define the geometry of the space.
In their work, Moreno and Koh tackled a “restricted” version of the three-body problem in which the spacecraft, one of the bodies, is so small as to have no effect on Jupiter or Europa, the other two bodies. Assuming the moon’s orbit was perfectly circular, the researchers further simplified the situation. Its circular orbit can be used as a stable backdrop to analyze the space probe’s trajectory. Since the motion of Jupiter and Europa can be easily described, the symplectic space only needs to account for the position and velocity of the spacecraft. The matching symplectic space is therefore six-dimensional as opposed to eighteen-dimensional. An orbit of the spacecraft through the planet-moon system is represented by a path that forms a loop in this six-dimensional system.
Koh was interested in instances where a spacecraft’s orbit would jump from one family to another when she got in touch with Moreno. Bifurcation points are the places where families of orbits come together. Many times, several families will come together at one location. For trajectory planners in particular, this makes them very helpful. “Knowing the bifurcation structure provides you with a road map as to where there are interesting trajectories that you should investigate,” stated Scheeres. Koh was interested in learning how to locate and anticipate bifurcation points.
Following his conversation with Koh, Moreno called in assistance from a few more geometers, including Otto van Koert of Seoul National University, Cengiz Aydin of Heidelberg University, and Urs Frauenfelder of the University of Augsburg. Symplectic geometry was used by Frauenfelder and van Koert to study the three-body problem for a considerable amount of time. They even discovered a possible new family of orbits. Though they have employed a wide range of mathematical tools, engineers designing spacecraft missions have recently become intimidated by the growing abstraction of symplectic geometry.
In the ensuing months, the four mathematicians and the engineer gradually became acquainted with each other’s areas of expertise. Speaking of language barriers, Moreno observed that working in an interdisciplinary setting takes time. But it begins to pay off once you’ve put in the patient work. “.
The Toolbox.
The group assembled several resources that they believe will be helpful to mission planners. The Conley-Zehnder index is one of the tools that can be used to identify whether two orbits are members of the same family. It is computed by looking at points that are near, but not on, the orbit that interests the researcher. For example, suppose that a spacecraft is impacted by the gravity of Europa and is in an elliptical orbit around Jupiter. Its new trajectory will roughly replicate the original orbit if you push it off course. Following its orbit around Jupiter, the new route will spiral back around the original orbit, returning to a slightly altered location. A gauge of the degree of spiraling is the Conley-Zehnder index.
Remarkably, the Conley-Zehnder index is a number related to the whole orbit and is independent of the precise manner in which the spacecraft is nudged. Additionally, for every orbit in the same family, it remains the same. Two different numbers indicate that the orbits are from different families if you compute the Conley-Zehnder index for two orbits.
Floer number is another tool that can provide hints about orbit families that have not yet been found. Let us consider the following scenario: a number of families collide at a bifurcation point when the energy reaches a certain value, and additional families split off from that bifurcation point when the energy exceeds a certain threshold. The bifurcation serves as the center of this web of families.
As a straightforward function of the Conley-Zehnder indices connected to each pertinent family, you can determine the Floer number linked to this bifurcation point. This function can be calculated for all families whose energy is greater than or equal to the bifurcation point, as well as for all families whose energy is only slightly less. A hint that hidden families are connected to your bifurcation point can be found if the two Floer numbers disagree.
Engineers can test their algorithms against our tools, according to Moreno. The new tools are not meant to replace the trajectory-finding methods that engineers have spent decades perfecting; rather, their main purpose is to assist engineers in understanding how families of orbits fit together and to encourage them to search for new families when necessary.
Moreno presented the work at a conference held in 2023 by the “Space Flight Mechanics Committee,” and he has maintained communication with engineers who study space trajectories, including those at JPL and Scheeres’ lab in Boulder. While Scheeres was well-versed in the symplectic approach to planetary motion, he felt mathematically inexperienced and appreciative of the mixing of fields. “Watching the mathematicians attempt to apply their knowledge to the engineering side was incredibly exciting,” he remarked. Scheeres’ team is currently developing a more intricate system with four bodies.
Womensfelder’s collaborator, Ed Belbruno, a trajectory planning consultant and former JPL orbital analyst, warns against taking the applications too literally. “While there are many trajectories that are really cool that can be found using a mathematical technique like symplectic geometry, it’s possible that very few, if any, of them satisfy the constraint” that a real mission might require, he said.
Moreno’s attention is focused on Saturn, even though the Clipper trajectories have already been determined to a large degree. Mission planners at JPL, who intend to send a spacecraft to Saturn’s moon Enceladus, have already heard about his research. Symplectic geometry should “become part of the standard space mission toolkit,” according to Moreno. “.
The initial narrative has been reposted with authorization from Quanta Magazine, an editorially autonomous periodical of the Simons Foundation. Its objective is to augment public comprehension of science by examining research advancements and patterns in mathematics, physics, and biology.